Lie group and lie algebra

A lie group is a differentiable manifold. An example of Lie group is the orthonormal matrix (ma trận trực giao) in R3x3, which is called SO(3).  Note that this group consists of the rotation matrices in Euclidean space. Another example of Lie group is the group of homogeneous transformation which is the special Euclidean group or SE(3).
Given rotation R  in SO(3) and translation b in R3, the homogeneous matrix is defined as follows
T = [R b;0 1]
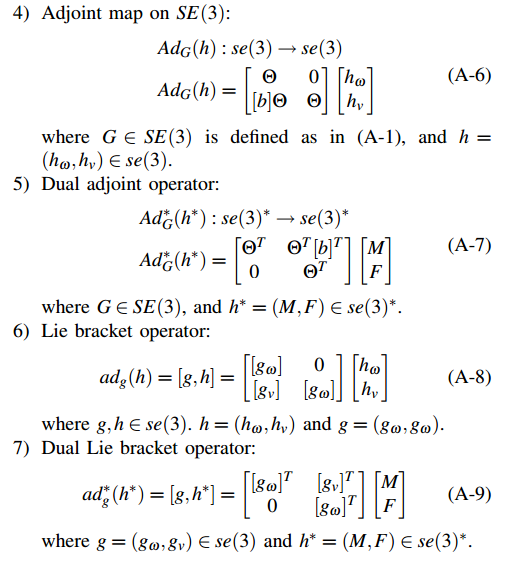
An important concept associated with each Lie group is the notation notation of Lie algebra.
The tangent space at the identity element of  a lie group is called the lie algebra for that group.
The Lie algebra of SO(3) and SE(3) are denote so(3) and se(3) respectively.
Let us define some notations and operations on Lie groups and Lie algebra: